GRAVITATIONAL FIELD OF A HEPTAGONAL
PLATE
INTRODUCTION
Gravity
is derived from the Latin word ‘gravitas’ meaning mass. The universal law of
gravitation was coined by Sir Isaac Newton. According to the law, any two
masses anywhere in the universe separated by a distance will attract each
other. This force of attraction is proportional to the product of their masses
and inversely proportional to square of the distance between them.
F = GM1M2/R2 (Eq. 1)
The
distance between two masses can be finite or infinite, which is why
gravitational force is referred to as long range force but is also the weakest
force among all the other fundamental forces. All objects that have mass will
attract other masses. This means that each mass has its own gravitational field
just like Earth. So this implies that all objects will attract each other since
they will have their own field. This is not evident on Earth since Earth’s
gravitational field outweighs all other mass’s field and hence all objects no
matter how massive are attracted toward the Earth. In this article the
gravitational field of a two dimensional Heptagonal plate will be determined and
points on the plate would be identified where the plate’s own gravity is strong
or weak.
ASSUMPTIONS
1. The
Heptagon is assumed to be a regular polygon.
2. The
thickness of the plate is negligible compared to its length and width.
3. The
plate is not under the influence of an external gravitational field.
4. The
plate is a homogeneous material.
5. All
the mass is assumed to be concentrated at the center.
CALCULATION
Consider
a Heptagonal plate of side length ‘a’ [m] and mass M [Kg]. First the center of
gravity of this plate will be determined, followed by the magnitude of the
gravitational field at points of interest.
Center of gravity
Fig 1 Heptagonal plate
The
center of gravity of Heptagon as determined from previous article is (x, y) ≡ (1.123a, h)
Points of interest and their
distances from center
Consider
two points namely Point A and Point B as shown in figure 1. Point A represents
the corner point while point B represents the mid-point of a side. All the
other points depicted in figure 1 are at the same distances as points A and B
are from the center.
Point A ≡ (0.623a, 0)
The
distance between Point A and C.G. can be calculated by the distance formula
Point B ≡ (1.123a, 0)
The
distance between Point B and C.G. can be calculated by the distance formula
Gravitational field
From
Eq. 1, the force of attraction between a mass and its own surface is given by,
g
= GM/R2
g
– Acceleration due to gravity of the mass (m/s2)
G
– Universal constant of gravitation
G
= 6.67*10-11 Nm2/Kg2
M
– Mass of the object (Kg)
R
– Distance between the centers of two masses (m)
G field at point A
The
gravitational field beyond the surface is obtained by adding the additional
distance,
d
– Distance between point A and any other point in space.
G field at point B
The
gravitational field beyond the surface is obtained by adding the additional
distance,
d
– Distance between point B and any other point in space.
CONCLUSION
Thus the magnitude of
gravitational field at two points on a Heptagonal plate was found successfully.
On comparing the gravitational acceleration values at point A and B it is
important to note that gravitational field is stronger at point B due to its
closer distance to the center while it is relatively weaker at point A due to
its greater distance from the center. This is true for every mid-point and
corner point on the Heptagon.

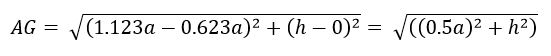





No comments:
Post a Comment