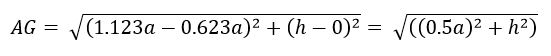GRAVITATIONAL
FIELD LINES OF AN ELLIPSE
INTRODUCTION
The force of gravity as
described by Sir Isaac Newton is a force of attraction. The gravitational force
acting on a body of mass ‘m’ is equal to the product of its mass ‘m’ and the
acceleration due to gravity acting on that body. Now Force is a vector quantity
since it is a product of mass [scalar] and acceleration [vector]. By definition
a scalar quantity can be represented by magnitude alone whereas a vector
quantity must be represented by magnitude and direction. Thus while solving a
vector quantity, it is important to obtain both magnitude and direction to
obtain complete information of the vector. Acceleration due to gravity being a
vector quantity has both magnitude and direction. The direction of gravitational
force is termed as gravitational field lines or orthogonal trajectories which
are always orthogonal [perpendicular] to the surface as explained in detail in
the calculation section. This article intends to determine the gravitational
field lines of a two dimensional elliptical plate.
ASSUMPTIONS
1. The plate has
negligible thickness hence is assumed to be two dimensional
2. The plate is
homogeneous in nature meaning composed of only one material
3. The plate is not under
the influence of an external gravitational field
4. The whole mass of the
plate is assumed to be concentrated in the center
CALCULATION
Consider a two
dimensional elliptical plate of major axis ‘a’ [m], minor axis ‘b’ [m] and mass
‘m’ [Kg]. Let the eccentricity of ellipse be around 1.2 which implies an
ellipse with major axis along x axis and a minor axis along y axis.
 |
| Fig .1 An Ellipse |
There is a 3 step
procedure to determine the field lines
Step1 Determine differential equation of
ellipse and its slope
The equation of a
regular Ellipse with major and minor axes ‘a’ and ‘b’ is,
Rearranging the above
equation,
Differentiating equation
(1) with respect to x,
Equation (3) represents
the slope of equation (1) [Ellipse]. Thus an orthogonal trajectory to the Ellipse
must have a slope that is negative inverse of the slope in equation (3).
Step2 Determine the slope of the
orthogonal trajectory
Thus the new slope or
the slope of the orthogonal trajectory which is a negative inverse of equation (3)
is
Step3 Determine equation of the
orthogonal trajectory by integration
To obtain the equation
of the orthogonal trajectory, integrate equation (4) by separating the
variables. Rearranging equation (4)
{‘k’ is a constant of
integration}
Equation (5) represents
the family of orthogonal trajectories of an ellipse. The orthogonal
trajectories which represent hyperbolas are pictorially represented in figure
2.
REPRESENTATION
The graph of an Ellipse
and its orthogonal trajectories are represented in Figure 2. The hyperbolas
which are orthogonal trajectories to the Ellipse appear emanating from the
Ellipse radially outward thereby satisfying the mathematical condition. Thus an
elliptical plate will have its gravitational field directed as hyperbolas.
Since an Ellipse is only symmetric about one axis x or y but not both, the
gravitational field lines are not equidistant from the center and hence the
magnitude of gravitational field is not the same at all points on the
circumference of Ellipse. From figure2, the field lines can be interpreted as
non-uniform since they are not parallel to each other. The non-uniformity stems
from the curvature of the curve [Ellipse].
 |
| Fig .2 Gravitational field lines of Ellipse |
EXPLANATION
The field lines to the
ellipse which are represented by hyperbolas do not appear to pass through the
center unlike the circle where straight lines did pass through the center. In
fact asymptotes of hyperbolas which are tangents to the hyperbolas do pass
through the center of ellipse. Asymptotes which are straight lines intersect
the hyperbolas at only one point. Since they intersect hyperbolas, their slope
is 0. Although hyperbolas themselves do not pass through the Ellipse’s center
of gravity their asymptotes do pass.
CONCLUSION
The final equation (5)
implies that the orthogonal trajectory of an Ellipse is family of hyperbolas.
It is important to note that the constant ‘2k’ can have infinite values hence
there are infinite orthogonal trajectories for a given shape in this case the Ellipse.